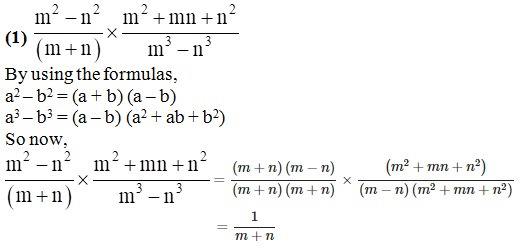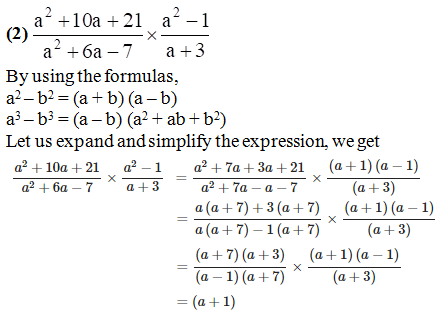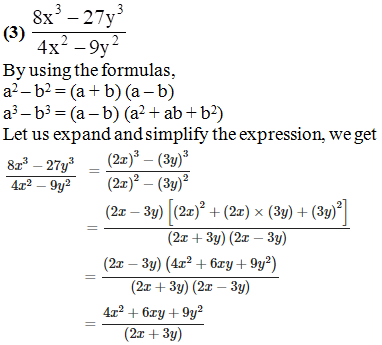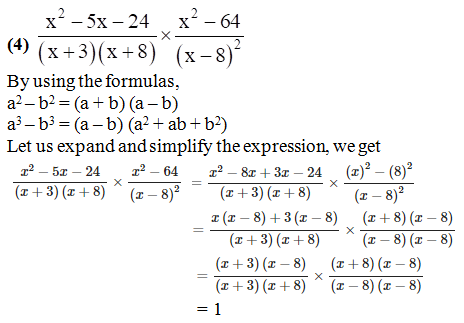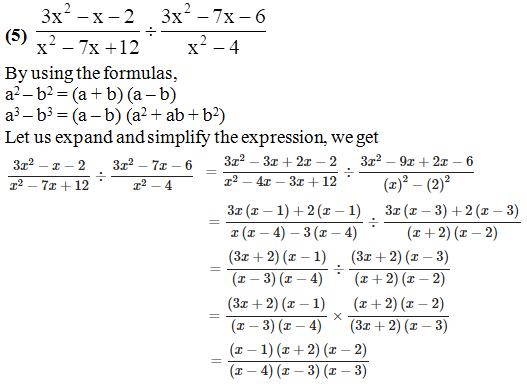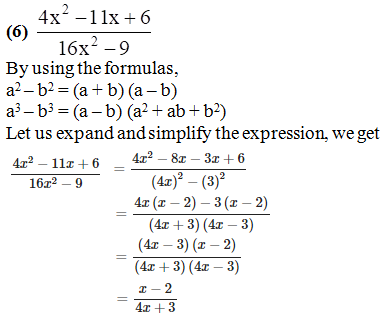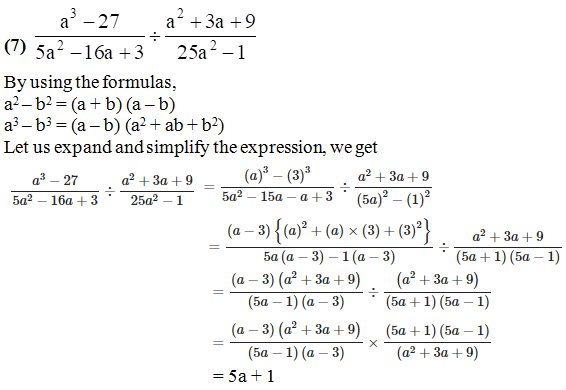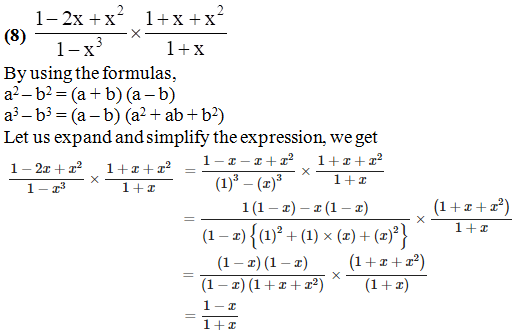Access answers to Maharashtra Board Solutions For Class 8 Maths Part 1 Chapter 6- Factorisation of Algebraic Expressions.

Practice set 6.1 PAGE NO: 30

1. Factorize:

(1) x² + 9x + 18

(2) x² – 10x + 9

(3) y² + 24y + 144

(4) 5y² + 5y – 10

(5) p² – 2p – 35

(6) p² – 7p – 44

(7) m² – 23m + 120

(8) m² – 25m + 100

(9) 3x² + 14x + 15

(10) 2x² + x – 45

(11) 20x² – 26x + 8

(12) 44x² – x – 3

Solution:

(1) x² + 9x + 18

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

x² + 9x + 18 = x² + 6x + 3x + 18

= x (x + 6) + 3(x + 6)

= (x + 6) (x + 3)

(2) x² – 10x + 9

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

x² – 10x + 9 = x² – 9x – x + 9

= x (x – 9) – 1(x – 9)

= (x – 9) (x – 1)

(3) y² + 24y + 144

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

y² + 24y + 144 = y² + 12y + 12y + 144

= y(y + 12) + 12(y + 12)

= (y + 12) (y + 12)

(4) 5y² + 5y – 10

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

5y² + 5y – 10 = 5(y² + y – 2) [By taking out the common factor 5]

= 5(y² + 2y – y – 2)

= 5[y(y + 2) – 1(y + 2)]

= 5 (p + 2) (y- 1)

(5) p² – 2p – 35

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

p² – 2p – 35 = p² – 7p + 5p – 35

= p(p – 7) + 5(p – 7)

= (p – 7) (p + 5)

(6) p² – 7p – 44

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

p² – 7p – 44 = p² – 11p + 4p – 44

= p(p – 11) + 4(p – 11)

= (p – 11) (p + 4)

(7) m² – 23m + 120

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

m² – 23m + 120 = m² – 15m – 8m + 120

= m (m – 15) – 8 (m – 15)

= (m – 15) (m – 8)

(8) m² – 25m + 100

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

m² – 25m + 100 = m² – 20m – 5m + 100

= m(m – 20) – 5(m – 20)

= (m – 20) (m – 5)

(9) 3x² + 14x + 15

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

3x² + 14x + 15 = 3x² + 9x + 5x + 15

= 3x(x + 3) + 5(x + 3)

= (x + 3) (3x + 5)

(10) 2x² + x – 45

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

2x² + x – 45 = 2x² + 10x – 9x – 45

= 2x(x + 5) – 9 (x + 5)

= (x + 5) (2x – 9)

(11) 20x² – 26x + 8

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

20x² – 26x + 8 = 2(10x² – 13x + 4) 10 × 4 = 40 [By taking out the common factor 2]

= 2(10x² – 8x – 5x + 4)

= 2[2x (5x – 4) – 1(5x – 4)]

= 2 (5x – 4) (2x – 1)

(12) 44x² – x – 3

On comparing with standard quadratic equation that is ax² + bx + c.

Let us simplify the given expression, we get

44x² – x – 3 = 44x² – 12x + 11x – 3

= 4x (11x – 3) + 1(11x – 3)

= (11x – 3) (4x + 1)

Practice set 6.2 PAGE NO: 31

1. Factorize:

(1) x³ + 64y³

(2) 125p³ + q³

(3) 125k³ + 27m³

(4) 2l³ + 432m³

(5) 24a³ + 81b³

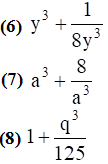
Solution:

(1) x³ + 64y³

We know that,

a³ + b³ = (a + b) (a² – ab + b²)

x³ + 64y³ = (x)³ + (4y)³

Here, a = x and b = 4y

Now, substituting in the above formula, we get

x³ + (4y)³ = (x + 4y) [x² – x(4y) + (4y)²]

= (x + 4y) (x² – 4xy + 16y²)

(2) 125p³ + q³

We know that,

a³ + b³ = (a + b) (a² – ab + b²)

125p³ + q³ = (5p)³ + q³

Here, a = 5p and b = q

Now, substituting in the above formula, we get

(5p)³ + q³ = (5p + q) [(5p)² – (5p)(q) + q²]

= (5p + q) (25p² – 5pq + q²)

(3) 125k³ + 27m³

We know that,

a³ + b³ = (a + b) (a² – ab + b²)

125k³ + 27m³ = (5k)³ + (3m)³

Here, a = 5k and b = 3m

Now, substituting in the above formula, we get

(5k)³ + (3m)³ = (5k + 3m) [(5k)² – (5k)(3m) + (3m)²]

= (5k + 3m) (25k² – 15km + 9m²)

(4) 2l³ + 432m³

We know that,

a³ + b³ = (a + b) (a² – ab + b²)

2l³ + 432m³ = 2 (l³ + 216m³ ) [By taking out the common factor 2]

= 2 (l³ + (6m)³ )

Here, a = l and b = 6m

Now, substituting in the above formula, we get

2 (l³ + (6m)³ ) = 2 {(l + 6m) [l² – l(6m) + (6m)² ]}

= 2 (l + 6m) (l² – 6lm + 36m² )

(5) 24a³ + 81b³

We know that,

a³ + b³ = (a + b) (a² – ab + b²)

24a³ + 81b³ = 3 [(2a)³ + (3b)³] [By taking out the common factor 3]

Here, a = 2a and b = 3b

Now, substituting in the above formula, we get

3 [(2a)³ + (3b)³] = 3 {(2a + 3b) [(2a)² – (2a)(3b) + (3b)²]}

= 3(2a + 3b) (4a² – 6ab + 9b²)

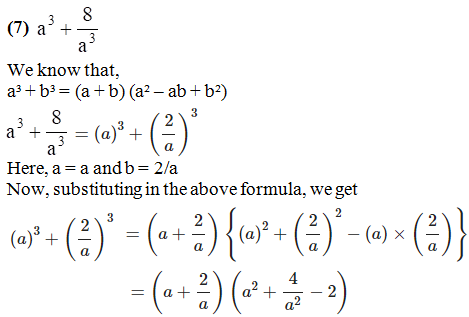
Practice set 6.3 PAGE NO: 32

1. Factorize:

(1) y³ – 27

(2) x³ – 64y³

(3) 27m³ – 216n³

(4) 125y³ – 1

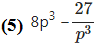
(6) 343a³ – 512b³

(7) 64x² – 729y²

Solution:

(1) y³ – 27

We know that,

a³ –b³ = (a –b) (a² + b² + ab)

y³ – 27 = y³ – (3)³

Here, a = y and b = 3

Now, substituting in the above formula, we get

y³ – (3)³ = (y – 3) [y² + y(3) + (3)2]

= (y – 3) (y² + 3y + 9)

(2) x³ – 64y³

We know that,

a³ –b³ = (a –b) (a² + b² + ab)

x³ – 64y³ = x³ – (4y)³

Here, a = x and b = 4y

Now, substituting in the above formula, we get

x³ – (4y)³ = (x – 4y) [x² + x(4y) + (4y)²]

= (x – 4y) (x² + 4xy + 16y²)

(3) 27m³ – 216n³

We know that,

a³ –b³ = (a –b) (a² + b² + ab)

27m³ – 216n³ = 27 (m³ – 8n³) [By taking out the common factor 27]

= 27 [m³ – (2n)³]

Here, a = m and b = 2n

Now, substituting in the above formula, we get

27 [m³ – (2n)³] = 27 {(m – 2n) [m² + m(2n) + (2n)²]}

= 27 (m – 2n) (m² + 2mn + 4n²)

(4) 125y³ – 1

We know that,

a³ –b³ = (a –b) (a² + b² + ab)

125y³ – 1= (5y)³ – 1³

Here, a = 5y and b = 1

Now, substituting in the above formula, we get

(5y)³ – 1³ = (5y – 1) [(5y)² + (5y)(1) + (1)²]

= (5y – 1) (25y² + 5y + 1)

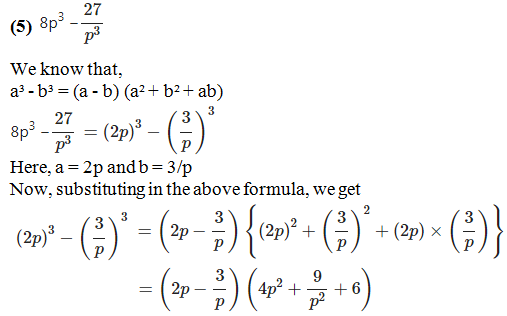
(6) 343a³ – 512b³

We know that,

a³ –b³ = (a –b) (a² + b² + ab)

343a³ – 512b³ = (7a)³ – (8b)³

Here, a = 7a and b = 8b

Now, substituting in the above formula, we get

(7a)³ – (8b)³ = (7a – 8b) [(7a)² + (7a)(8b) + (8b)²]

= (7a – 8b) (49a² + 56ab + 64b²)

(7) 64x² – 729y²

We know that,

a³ –b³ = (a –b) (a² + b² + ab)

64x² – 729y² = (4x)³ – (9y)³

Here, a = 4x and b = 9y

Now, substituting in the above formula, we get

(4x)³ – (9y)³ = (4x – 9y) [(4x)² + (4x) (9y) + (9y)²]

= (4x – 9y) (16x² + 36xy + 81y²)

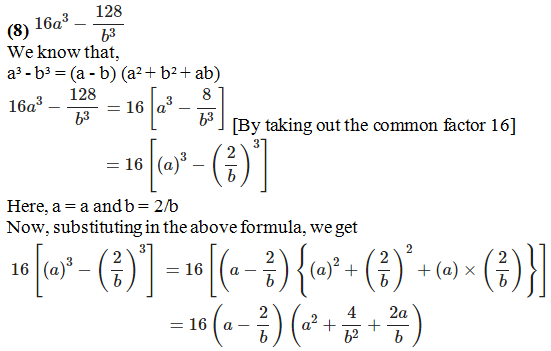
2. Simplify:

(1) (x + y)³ – (x – y)³

(2) (3a + 5b)³ – (3a – 5b)³

(3) (a + b)³ – a³ – b³

(4) p³ – (p + 1)³

(5) (3xy – 2ab)³ – (3xy + 2ab)³

Solution:

(1) (x + y)³ – (x – y)³

Let us consider,

Here, a = x + y and b = x – y

By using the formula,

[a³ – b³ = (a – b) (a² + ab + b²)]

Let us simplify the given expression, we get

(x + y)³ – (x – y)³ = [(x + y) – (x – y)] [(x + y)² + (x + y) (x – y) + (x – y)]

= (x + y – x + y) [(x² + 2xy + y²) + (x² – y²) + (x² – 2xy + y²)]

= 2y(x² + x² + x² + 2xy – 2xy + y² – y² + y²)

= 2y (3x² + y²)

= 6x²y + 2y³

(2) (3a + 5b)³ – (3a – 5b)³

Let us consider,

Here, a = 3a + 5b and b = 3a – 5b

By using the formula,

[a³ – b³ = (a – b) (a² + ab + b²)]

Let us simplify the given expression, we get

(3a + 5b)³ – (3a – 5b)³ = [(3a + 5b) – (3a – 5b)] [(3a + 5b)² + (3a + 5b) (3a – 5b) + (3a – 5b)²]

= (3a + 5b – 3a + 5b) [(9a² + 30ab + 25b²) + (9a² – 25b²) + (9a² – 30ab + 25b²)]

= 10b (9a² + 9a² + 9a² + 30ab – 30ab + 25b² – 25b² + 25b²)

= 10b (27a² + 25b²)

= 270a²b + 250b³

(3) (a + b)³ – a³ – b³

By using the formula,

[a³ + b³ = a³ + b³ + 3a² b + 3ab² ]

By substituting in the above equation, we get

(a + b)³ – a³ – b³ = a³ + 3a²b + 3ab² + b³ – a³ – b³

= 3a²b + 3ab²

(4) p³ – (p + 1)³

By using the formula,

[a³ + b³ = a³ + b³ + 3a² b + 3ab² ]

By substituting in the above equation, we get

p³ – (p + 1)³ = p³ – (p³ + 3p² + 3p + 1)

= p³ – p³ – 3p² – 3p – 1

= – 3p² – 3p – 1

(5) (3xy – 2ab)³ – (3xy + 2ab)³

Let us consider,

Here, a = 3xy – 2ab and b = 3xy + 2ab

By using the formula,

[a³ – b³ = (a – b) (a² + ab + b²)]

Let us simplify the given expression, we get

(3xy – 2ab)³ – (3xy + 2ab)³ = [(3xy – 2ab) – (3xy + 2ab)] [(3xy – 2ab)² + (3xy – 2ab) (3xy + 2ab) + (3xy + 2ab)²]

= (3xy – 2ab – 3xy – 2ab) [(9x²y² – 12xyab + 4a²b²) + (9x²y² – 4a²b²) + (9x²y² + 12xyab + 4a²b²)]

= (- 4ab) (9x²y² + 9x²y² + 9x²y² – 12xyab + 12xyab + 4a²b² – 4a²b² + 4a²b²)

= (- 4ab) (27 xy² + 4a²b²)

= -108x²y²ab – 16a³b³

Practice set 6.4 PAGE NO: 33

1. Simplify:

Solution: