The Class 8 is an important year in a student’s life and Maharashtra State Board Maths is one of the subjects that require dedication, hard work, and practice. It’s a subject where you can score well if you are well-versed with the concepts, remember the important formulas and solving methods, and have done an ample amount of practice. Worry not! Home Revise is here to make your Class 8 journey even easier. It’s essential for students to have the right study material and notes to prepare for their board examinations, and through Home Revise, you can cover all the fundamental topics in the subject and the complete Maharashtra State Board Class 8 Maths Book syllabus.
Practice Set 17.1 Page No: 116
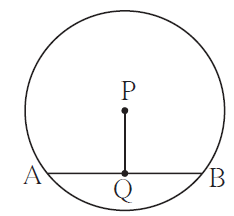
1. In a circle with centre P, chord AB is drawn of length 13 cm, seg PQ ⊥ chord AB, then find l (QB).
Solution:
Given,
seg PQ ⊥ chord AB and l(AB) = 13 cm
Now,
l(QB) = ½ l(AB
[As the perpendicular drawn from the centre of a circle to its chord bisects the chord]⇒ l(QB) = ½ x 13
Thus, l(QB) = 6.5 cm
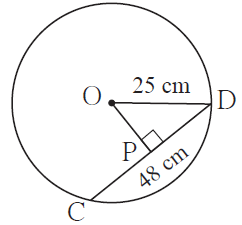
2. Radius of a circle with centre O is 25 cm. Find the distance of a chord from the centre if length of the chord is 48 cm.
Solution:
Given,
seg OP ⊥ chord CD and l(CD) = 48 cm
Radius of circle = 25 cm, so OD = 25 cm
Now,
l(PD) = ½ l(CD)
[Perpendicular drawn from the centre of a circle to its chord bisects the chord]l(PD) = ½ x 48
⇒ l(PD) = 24 cm … (i)
In ∆OPD, we have
m ∠OPD = 90°
So, by Pythagoras theorem
[l(OD)]² = [l(OP)]² + [l(PD)]²(25)² = [l(OP)]² + (24)² [From (i)]
(25)² – (24)² = [l(OP)]²
(25 + 24) (25 – 24) = [l(OP)]² [Since, a² – b² = (a + b) (a – b)]
49 x 1 = [l(OP)]²
[l(OP)]² = 49∴l(OP) = √49 = 7 cm [Taking square root of both sides]
Thus, the distance of the chord from the centre of the circle is 7 cm.
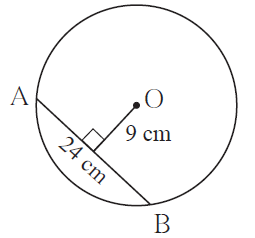
3. O is centre of the circle. Find the length of radius, if the chord of length 24 cm is at a distance of 9 cm from the centre of the circle.
Solution:
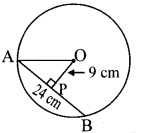Let seg OP ⊥ chord AB
l(AB) = 24 cm and l(OP) = 9 cm
Now,
l(AP) = ½ l(AB)
[Perpendicular drawn from the centre of a circle to its chord bisects the chord]l(AP) = ½ x 24
⇒ l(AP) = 12 cm …(i)
In ∆OPA, we have
m ∠OPA = 90°
So, by Pythagoras theorem
[l(AO)]² = [l(OP)]² + [l(AP)]²[l(AO)]² = (9)² + (12)² [From (i)]= 81 + 144 = 225
⇒ l(AO) = √225 = 15 cm [Taking square root of both sides]
Thus, the length of radius of the circle is 15 cm.
4. C is the centre of the circle whose radius is 10 cm. Find the distance of the chord from the centre if the length of the chord is 12 cm.
Solution:
Let’s consider a circle of radius 10 cm and centre C.
And, seg AB be the chord of the circle
Now, draw seg CD ⊥ chord AB, l(AB) = 12 cm and l(AC) = 10 cm.
l(AD) = ½ l(AB)
[Perpendicular drawn from the centre of a circle to its chord bisects the chord]l(AD) = ½ x 12
⇒ l(AD) = 6 cm …(i)
So,
In ∆ACD, m ∠ADC = 90°
By Pythagoras theorem,
[l(AC)]² = [l(AD)]² + [l(CD)]²(10)² = (6)² + [l(CD)]² [From (i) and l(AC) = 10 cm]
(10)² – (6)² = [l(CD)]²
100 – 36 = [l(CD)]²
64 = [l(CD)]²
⇒ l(CD) = √64 = 8 cm [Taking square root of both sides]
Thus, the distance of the chord from the centre of the circle is 8 cm.
Practice Set 17.2 Page No: 118
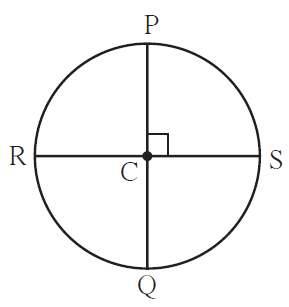
1. The diameters PQ and RS of the circle with centre C are perpendicular to each other at C. State, why arc PS and arc SQ are congruent. Write the other arcs which are congruent to arc PS.
Solution:
Given, diameter PQ ⊥ diameter RS
So, we have
m ∠PCS = m ∠SCQ = m ∠PCR = m ∠RCQ = 90°
We know that, the measure of the angle subtended at the centre by an arc is the measure of the arc.
So,
m (arc PS) = m ∠PCS = 90° … (i)
m (arc SQ) = m ∠SCQ = 90° … (ii)
From (i) and (ii), we see that
m (arc PS) = m (arc SQ)
Hence, arc PS ≅ arc SQ
[If the measures of two arcs of a circle are same, then the two arcs are congruent]Similarly,
m (arc PR) = m ∠PCR = 90° … (iii)
m (arc RQ) = m ∠RCQ = 90° … (iv)
Hence, from (i), (iii) and (iv) we have
m (arc PS) = m (arc PR) = m (arc RQ)
∴ arc PS ≅ arc PR ≅ arc RQ
[If the measures of two arcs of a circle are same, then the two arcs are congruent]Thus, arc PR and arc RQ are congruent to arc PS.
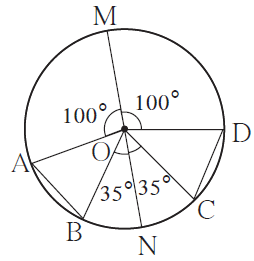
2. In the adjoining figure O is the centre of the circle whose diameter is MN. Measures of some central angles are given in the figure. Hence find the following
(1) m ∠ AOB and m ∠ COD
(2) Show that arc AB ≅ arc CD.
(3) Show that chord AB ≅ chord CD
Solution:
(1) Given,
Seg MN is the diameter of the circle and m ∠AOM = 100°, m ∠BON = 35°, m ∠DOM = 100° and m ∠CON = 35°
Now,
m ∠AOM + m ∠AON = 180° [Angles in a linear pair]
m ∠AOM + (m ∠AOB + m ∠BON) = 180° [Angle addition property]
100° + m ∠AOB + 35° = 180°
m ∠AOB + 135° = 180°
m ∠AOB = 180°- 135°
⇒ m ∠AOB = 45° … (i)
Also, we have
m ∠DOM + m ∠DON = 180° [Angles in a linear pair]
m ∠DOM + (m ∠COD + m ∠CON) = 180° [Angle addition property]
100° + m ∠COD + 35°= 180°
m ∠COD + 135° = 180°
m ∠COD = 180°- 135°
⇒ m ∠COD = 45° … (ii)
(2) Now, m (arc AB) = m ∠AOB = 45° [From (i)]
And, m (arc DC) = m ∠DOC = 45° [From (ii)]
So, from (i) and (ii)
m (arc AB) = m (arc DC)
Hence,
arc AB ≅ arc CD
[If the measures of two arcs of a circle are same, then the two arcs are congruent](3) We have, arc AB ≅ arc CD [Proved above]
Hence, as the chords corresponding to congruent arcs are congruent
chord AB ≅ chord CD.