Access answers to Maharashtra Board Solutions For Class 8 Maths Part 2 Chapter 14 Compound interest.

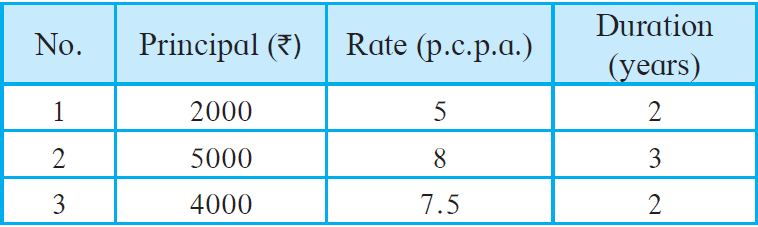
Practice Set 14.1 Page No: 90

1. Find the amount and the compound interest.

Solution:

(1) Here we have, P = ₹ 2000; R = 5 %; N = 2 years

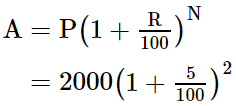
= 2000 (105/100)²

= 2000 (21/20)²

= 2205

So, the compound interest after 2 years will be

I = Amount – Principal

= Rs 2205 – Rs 2000

= Rs 205

Therefore, the amount is Rs 2205 and compound interest is Rs 205.

(2) Here we have, P = ₹ 5000; R = 8 %; N = 3 years

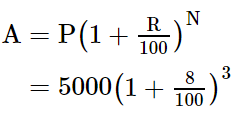
= 5000 (108/100)³

= 5000 (27/25)³

= 6298.56

So, the compound interest after 3 years will be

I = Amount – Principal

= Rs 6298.56 – Rs 5000

= Rs 1298.56

Therefore, the amount is Rs 6298.56 and compound interest is Rs 1298.56.

(3) Here we have, P = ₹ 4000; R = 7.5 %; N = 2 years

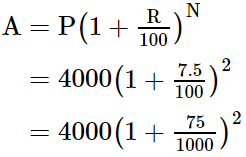
= 4000(1075/1000)²

= 4000(43/40)²

= 4622.50

So, the compound interest after 2 years will be

I = Amount – Principal

= Rs 4622.50 – Rs 4000

= Rs 622.50

Therefore, the amount is Rs 4622.50 and compound interest is Rs 622.50.

2. Sameerrao has taken a loan of Rs 12500 at a rate of 12 p.c.p.a. for 3 years. If the interest is compounded annually then how many rupees should he pay to clear his loan?

Solution:

Here we have, P = ₹ 12500; R = 12 %; N = 3 years

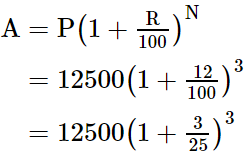
= 12500 (28/25)³

= 17561.60

Therefore, Sameerrao should pay an amount of Rs 17561.60 to clear his loan.

3. To start a business Shalaka has taken a loan of Rs 8000 at a rate of 10 ½ p.c.p.a. After two years how much compound interest will she have to pay?

Solution:

Here we have, P = ₹ 8000; R = 101212 %; N = 2 years

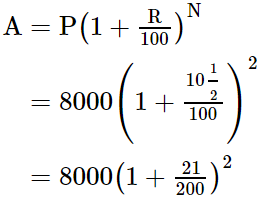
= 8000 (221/200)²

= 9768.20

So, the compound interest after 2 years will be

I = Amount – Principal

= Rs 9768.20 – Rs 8000

= Rs 1768.20

Therefore, Shalaka will have to pay a compound interest of Rs 1768.20 after 2 years.

Practice Set 14.2 Page No: 93

1. On the construction work of a flyover bridge there were 320 workers initially. The number of workers were increased by 25% every year. Find the number of workers after 2 years.

Solution:

From the given information,

Here we have, P = Number of workers initially = 320

A = Number of workers after 2 years

R = Rate of increase of number of workers per year = 25 %

N = 2 years

So,

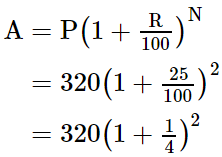
= 320 (5/4)²

= 500

Therefore, the number of workers after 2 years is 500.

2. A shepherd has 200 sheep with him. Find the number of sheeps with him after 3 years if the increase in number of sheeps is 8% every year.

Solution:

From the given information,

Here we have, P = Number of sheeps initially = 200

A = Number of sheeps after 3 years

R = Rate of increase of number of sheeps per year = 8 %

N = 3 years

So,

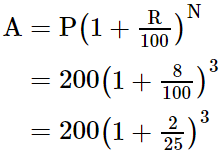
= 200 (27/25)³

= 251.94 ~ 252

Therefore, the number of sheeps after 3 years is 252.

3. In a forest there are 40,000 trees. Find the expected number of trees after 3 years if the objective is to increase the number at the rate 5% per year.

Solution:

From the given information,

Here we have, P = Number of trees initially = 40,000

A = Number of trees after 3 years

R = Rate of increase of number of trees per year = 5 %

N = 3 years

So,

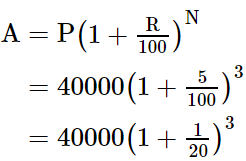
= 40000 (21/20)³

= 46305

Therefore, the expected number of trees after 3 years is 46,305.

4. The cost price of a machine is 2,50,000. If the rate of depreciation is 10% per year find the depreciation in price of the machine after two years.

Solution:

From the given information,

Here we have, P = Cost price of the machine = 2,50,000

A = Cost price after 2 years

I = Depreciation in price after 2 years

R = Rate of depreciation = 10 %

N = 2 years

So,

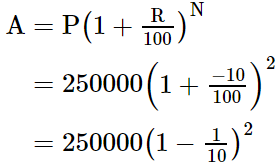
= 250000(9/10)²

= 202500

Also, the interest is

I = P − A

= Rs 250000 – Rs 202500

= Rs 47500

Therefore, the depreciation in price of the machine after two years is Rs 47,500.

5. Find the compound interest if the amount of a certain principal after two years is Rs 4036.80 at the rate of 16 p.c.p.a.

Solution:

From the given information,

Here we have,

P = Principal

A = ₹ 4036.80

I = Compound Interest

R = 16 %

N = 2 years

So,

Now, the interest is

I = A − P

= 4036.80 − 3000

= 1036.80

Therefore, the compound interest is ₹ 1036.80

6. A loan of Rs 15000 was taken on compound interest. If the rate of compound interest is 12 p.c.p.a. find the amount to settle the loan after 3 years.

Solution:

From the given information,

Here we have, P = Principal = ₹ 15000

A = Amount

R = 12 %

N = 3 years

So, the amount

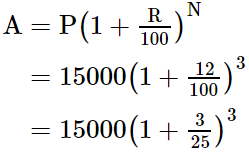
= 15000 (28/25)³

= 21073.92

Therefore, the amount to settle the loan is ₹ 21073.92

7. A principal amounts to Rs 13924 in 2 years by compound interest at 18 p.c.p.a. Find the principal.

Solution:

From the given information,

Here we have, P = Principal

A = ₹ 13924

R = 18 %

N = 2 years

We know that, the amount is

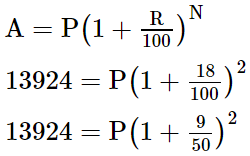
13924 = P (59/50)²

P = (13924 x 50 x 50)/ (59 x 59)

⇒ P = 10000

Therefore, the principal is Rs 10000.

8. The population of a suburb is 16000. Find the rate of increase in the population if the population after two years is 17640.

Solution:

From the given information,

Here we have, P = Population of a suburb = 16000

A = Population after two years =17640

R = R %

N = 2 years

We know that, the amount is given by

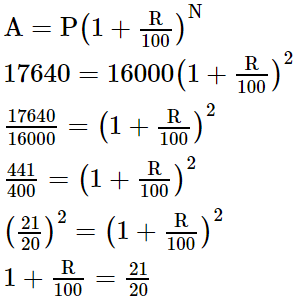
R/100 = 21/20 – 1

R/100 = 1/20

⇒ R = 5

Therefore, the rate of increase in the population is 5 p.c.p.a.

9. In how many years Rs 700 will amount to Rs 847 at a compound interest rate of 10 p.c.p.a.

Solution:

From the given information,

Here we have, P = Principal = ₹ 700

A = Amount = ₹ 847

R = 10 %

N = N years

We know that, the amount is given by

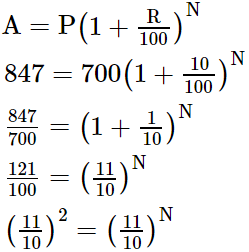
⇒ N = 2

Therefore, the numbers of years is 2 years.

10. Find the difference between simple interest and compound interest on Rs 20000 at 8 p.c.p.a.

Solution:

Given,

P = Principal = ₹ 20000

R = 8 %

As the time is not given, the question is solved by taking time as 2 years, because simple interest and compound interest will be same for one year.

N = 2 years

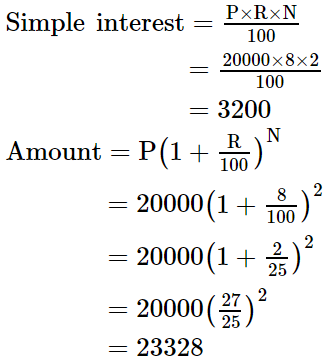
So, the compound interest = Rs 23328 – Rs 20000 = Rs 3328

Now, comparing compound interest and simple interest we have

⇒ Compound interest – Simple interest = Rs 3328 – Rs 3200 = Rs 128

Therefore, the difference between simple interest and compound interest is Rs 128.